Read more
Earth Day: Pogo and our responsibility
Read more
Carbon Content in Dwarf Stars
Explore how white dwarfs contribute essential carbon to the cosmos, impacting debates about its origins in the Milky Way.
Read more
What we can say about Google and 2024 Nobel Prizes
2024 Nobel Prizes spotlight breakthroughs in AI, neural networks, and protein folding advancements.I apologize for the delayed publication of this post, but due to the problems with the security certificate that I was writing last week, I preferred to leave this article on hold, so I'm recovering it now.
Read more
The Abel Prize 2025: Masaki Kashiwara
Masaki Kashiwara wins the 2025 Abel Prize for groundbreaking work in algebraic analysis, D-modules theory, and crystal bases in representation theory.Continue reading
The All-New, All-Different Doc Madhattan
After a long search I stumbled upon Hashnode, which also allows you to receive posts directly in your email inbox by subscribing to the newsletter, a very useful tool.
I then moved the archive of posts to the new blog by first exporting and then converting them to markdown using this converter. The transition was not error-free and I had to manually insert a couple of posts, while this one is completely absent because the video inside, after more than ten years, is no longer available.
At the end of the transfer, I discovered that some comments and discussions under the posts were promoted to real posts on Hashnode. I deleted the ones I found, as well as the drafts that had become public when the backup was uploaded.
The mathematical equations, on the other hand, seem to have been interpreted correctly, apart from a few things that I hope to fix as I discover them, but with an archive of over 400 posts, your help could be useful, so feel free to point out things that are wrong in the comments of this new introductory post.
I will not stop using the blog on Fields of Science, however: in this case I will publish an excerpt of the post with a link to the full article on Hashnode: I do not want to forget the readers who continue to follow that platform, even if I invite them and all new readers to update their rss readers, blogrolls and subscribe to the newsletter!
Doc Madhattan is back and I hope to be able to publish a little more regularly than in previous years here on Hashnode!
Geometric model of particles: a didactical approach
Working on Guido's particle model, however, has always been a worry of mine, so a couple of years ago I proposed to him to try to develop a didactic formulation of the model that could be used to bring elementary particles not only to university, but also to high school. From that idea, although my contributions to the writing were minimal, a triptych of articles came out, of which you can find the links below, and which received a particular review that made me very happy:
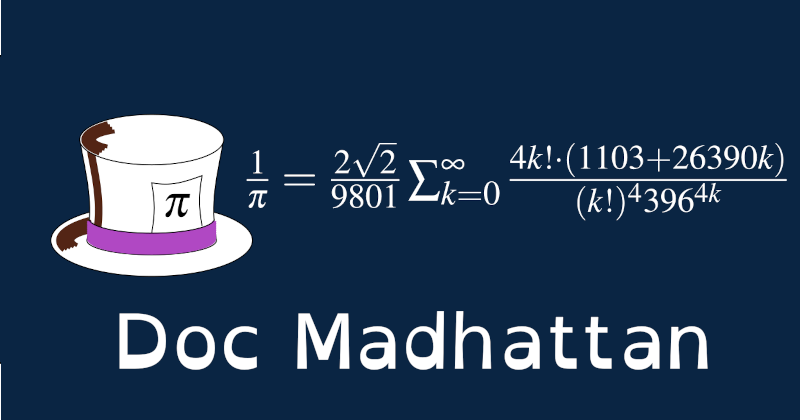
The Authors propose a didactic model representative of the particles described of the Standard Model. In this approach, particles result to be geometric forms corresponding to geometric structures of coupled quantum oscillators. An in-depth phenomenology of particles surfaces and this seems fully compatible with that of the Standard Model. Consequently, it is possible to calculate the mass of Higgs's Boson and the mass of the pair "muon and muonic neutrino" in "geometrical" sense. Via this geometric approach, it seems also possible to solve crucial aspects of the Standard Model. as the neutrinos’ oscillations and the intrinsic chirality of the neutrino and antineutrino. The paper is very interesting and deserves immediate publication in JHEPGC.I don't consider the work finished and indeed I would like to be able to bring these ideas into practice in schools. For now I'm happy to share this happiness here on the blog.
The Geometric Model of Particles: An Original Didactic about Standard Model -> The Quarks | Nucleons and K-Mesons | Leptons and Bosons
Leonardo's gears
The Madrid Codices I and II are two collections of Leonardo da Vinci's manuscripts found in a collection in the National Library of Madrid at the end of the 1960s. In particular, the Madrid Codex I consists of 382 pages of notes accompanied by something like 1600 between sketches and drawings and addresses a problem for which Leonardo is in some way particularly known as an engineer and designer: gears.
Leonardo's starting point is the study of friction. This is a force that opposes motion, but thanks to its opposition it is possible for us to walk without slipping or losing balance. As we all know today, however, the intensity of the frictional force depends on the surfaces that are in contact with each other, on how smooth or rough they are, which is independent of the area in contact and which can be reduced by using, for example, a lubricant or cylinders. All this, however, was already known to Leonardo, as it is possible to observe from the reading of the Madrid Code I. Furthermore, it is always Leonardo who introduced the concept of friction coefficient, defining it as the ratio between the force required to slide two surfaces horizontally on top of each other and the pressure between the two surfaces. Leonardo also estimated the value of this friction coefficient in 1/4, consistent with the materials best known to the florentine and with which he could carry out experiments (wood on wood, bronze on steel, etc.)(1).
At this point Leonardo is ready to develop a series of gears capable of carrying mechanical energy and producing motion, minimizing friction with the use of spheres and cylinders, as can be seen from his numerous drawings. In particular, however, it is Leonardo's mechanical use of two particular geometric shapes that is striking, because it anticipates their actual adoption by centuries: the epicloidal teeth and the globoidal gear.
Leonardo's starting point is the study of friction. This is a force that opposes motion, but thanks to its opposition it is possible for us to walk without slipping or losing balance. As we all know today, however, the intensity of the frictional force depends on the surfaces that are in contact with each other, on how smooth or rough they are, which is independent of the area in contact and which can be reduced by using, for example, a lubricant or cylinders. All this, however, was already known to Leonardo, as it is possible to observe from the reading of the Madrid Code I. Furthermore, it is always Leonardo who introduced the concept of friction coefficient, defining it as the ratio between the force required to slide two surfaces horizontally on top of each other and the pressure between the two surfaces. Leonardo also estimated the value of this friction coefficient in 1/4, consistent with the materials best known to the florentine and with which he could carry out experiments (wood on wood, bronze on steel, etc.)(1).
At this point Leonardo is ready to develop a series of gears capable of carrying mechanical energy and producing motion, minimizing friction with the use of spheres and cylinders, as can be seen from his numerous drawings. In particular, however, it is Leonardo's mechanical use of two particular geometric shapes that is striking, because it anticipates their actual adoption by centuries: the epicloidal teeth and the globoidal gear.
Subscribe to:
Posts (Atom)